5.10: Reconstituted Medication
- Page ID
- 44545
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)In the previous section, we calculated medication doses that were provided in a liquid form in a given concentration. Medications are also commonly supplied in dry form, such as powders or crystals, that must be reconstituted with fluid before they are administered parenterally by injection. Reconstitution is the process of adding a liquid diluent to a dry ingredient to make a specific concentration of liquid. See Figure \(\PageIndex{1}\)[1] for an example of a vial of medication that requires reconstitution. When reconstituting medications, it is important to follow the reconstitution instructions carefully so the medication is prepared in the correct concentration. When calculating the dosage of reconstituted medication to administer to the patient, the amount of fluid used to dilute the medication must also be considered. Let’s practice using dimensional analysis to determine how much of a reconstituted medication should be administered.
Practice Problem: Reconstituted Medication
Patient Information:
Name: Liam Vang, DOB: 04/04/19xx, Age 8, Allergies: NKDA, Weight: 60 kg
Provider Order: Cefazolin 500 mg IM every 8 hours
Medication Supplied: See Figure \(\PageIndex{2}\)[2] for the drug label of the medication as it is supplied.
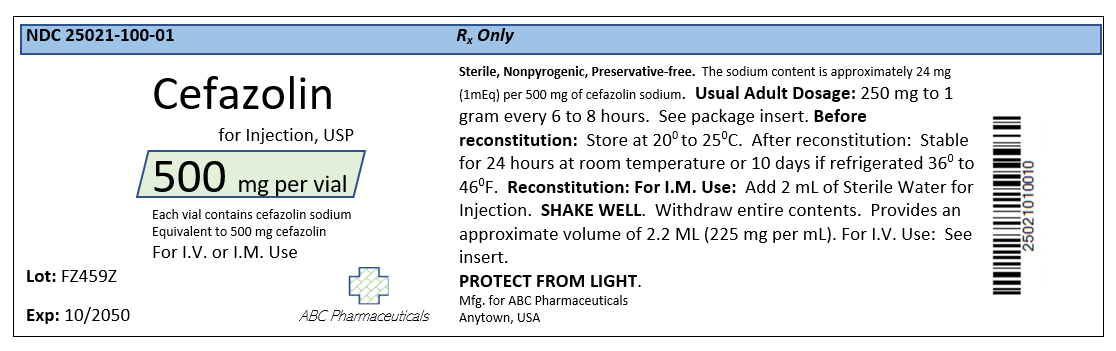
- Start by reading the order and the drug label. Liam has an order to receive 500 mg of Cefazolin. The vial of medication in powder form states there are 500 mg per vial. The powder must be reconstituted before it can be administered. The reconstitution instructions on the label state to add 2 mL of sterile water to the vial to reconstitute the powder into a liquid form for injection. The label states that after the powder is reconstituted with the 2 mL of diluent, the concentration of fluid will be 225 mg/mL. See a close-up image of reconstitution instructions in Figure \(\PageIndex{3}\).[3]
When setting up the problem, we need to identify the correct information to include in the equation. There are several numbers we may be tempted to try to incorporate into our equation, such as 500 mg per vial, 2 mL diluent, and approximate volume of 2.2 mL. These are numbers specific to the reconstitution process. However, keep in mind that our final goal is to calculate the number of mL of fluid to administer after the medication is reconstituted, so this will be the goal unit. The other piece of important information that the drug label states is that the reconstituted medication will provide a concentration of 225 mg/mL.
2. Start by identifying the goal unit for which you are solving, which is mL to administer as an injection:
\[ mL~=~? \]
3. Set up the first fraction by matching the numerator to the goal unit of mL. In this problem, we know from the drug label that the known concentration of the reconstituted medication is 225 mg per mL, so add 1 mL to the numerator and 225 mg to the denominator:
\[ mL=\frac{1~mL}{225~mg} \]
4. Set up the second fraction with mg in the numerator with the intent to cross off mg diagonally. Look at the given information to determine how it relates to mg. The order tells us to give Liam 500 mg of the medication. Plug in 500 in the numerator of the second fraction with one in the denominator so that mg will cross off diagonally:
\[ mL=\frac{1~mL}{225~mg}~~\times~~\frac{500~mg}{1} \]
5. Cross off units diagonally. Multiply across the numerators and the denominators, and then divide the final fraction for the answer in mL:
\[ mL=\frac{1~mL}{225~\cancel{mg}}~~\times~~\frac{500~\cancel{mg}}{1}~~=~~\frac{500~mL}{225}~=~2.2222~mL \]
6. Because the patient is a child, round to hundredth for the final answer: 2.22 mL
Please practice medication reconstitution calculations with the following interactive learning activity.
Query \(\PageIndex{1}\)
- “Zevtera 1Vial UK(3)(1) (29608654394).jpg” by Mohamd Ghani is licensed under CC BY-SA 2.0↵
- “Cefazolin Label Fig. 9.PNG" by Jody Myhre-Oechsle, Chippewa Valley Technical College, Open RN is licensed under CC BY 4.0↵
- “Reconstitution Instructions.png" by Jody Myhre-Oechsle, Chippewa Valley Technical College, Open RN is licensed under CC BY 4.0↵
- Southwest Tech Math/Science Center. (2018, April 25). Entry-level drug calculations for nursing students part 3 – Pharmacology, nursing math*. [Video]. YouTube. All rights reserved. Video used with permission. https://youtu.be/g9nqo-aZuHE↵
- RegisteredNurseRN. (2015, October 7). Dosage calculations made easy | Reconstitution calculation medication problems nursing students (10). [Video]. YouTube. All rights reserved. Video used with permission. https://youtu.be/TK3ZAaMuhYk↵